Mothra. A new Kaiju star in the sky and dark matter

Posted on by chemadiegor
Mothra is believed to be a binary system, with a cold (red) star and a hot (blue) star orbiting around the common center of mass. The red companion is observed to be varying with time, a common situation in this type of systems.

Monster stars are giant superluminous stars, tens of thousands or hundreds of thousands brighter than the Sun. Despite their superb luminosity, with the most powerfull telescopes they can only be seen in our Galaxy or in galaxies nearby. However, astronomers can rely on a trick offered by nature to see these stars much, much farther away. As predicted by general relativity, space can bend in the presence of very massive objects. Light traveling through that warped space bends as well, and can be focused into our telescopes in the same way a piece glass that is shaped into a lens can focus light into a focal point.

The most massive objects known capable of bending space are galaxy clusters, with masses up to thousands of times the mass of our own Galaxy. If a telescope points towards one of these massive galaxy clusters, objects that are very far away and right behind the cluster can be magnified, the same way a lens at the end of a telescope would magnify distant objects. The combined effect of a human made telescope looking through the natural telescope that is a galaxy cluster is similar to a larger telescope, hence allowing to see objects that are too faint to be observed directly without the aid of these natural telescopes. The magnification provided by these natural telescopes (galaxy clusters) is not uniform, and there is a very narrow region around the center of the galaxy cluster where the magnification factor can reach several thousands. These narrow regions are known as critical curves and objects that are observed near these curves are magnified by very large factors. With magnification factors ~1000 provided by these natural lenses, a telescope such as the JWST (with a mirror size of ~6 m) observing a star near a critical curve becomes the equivalent of a giant telescope with a mirror size of ~200 meters in diameter. That is, 20 times larger than the largest telescope on Earth (The Spanish GTC in the Canary Islands). With such giant telescopes, astronomers can see very luminous individual stars at very large distances, provided these stars happen to be observed close enough to a critical curve.

Several stars have been observed this way, and in previous posts we have discussed a few of them (Earendel, Godzilla). In a recent work, we present a new Kaiju star named Mothra, which is specially interesting because it can be used to study models of dark matter (DM), arguably, the most mysterious substance in the universe (with the permission of space itself). When observing lensed stars with very large magnification factors, we often see a double image of the star twice, one on each side of the critical curve but very close to it. One of the star has positive parity (that is, it represents a direct image of the true image), and the other has a negative parity (that is, it appears as a reflection of the true image). For stars, we can not directly observe the parity since we only measure their fluxes, and we can not resolve the star even with magnification factors of several thousands. However, the parity of the image can be easily established from a model of the galaxy cluster. In the case of Mothra, we only see one of the two expected images. This is not unusual for images that have positive parity, since images of stars with negative parity are often demagnifed by tiny lenses in the galaxy cluster. This tiny lenses are stars in the galaxy cluster that introduce small imprefections on the otherwise nearly perfectly smooth gravitational lens.

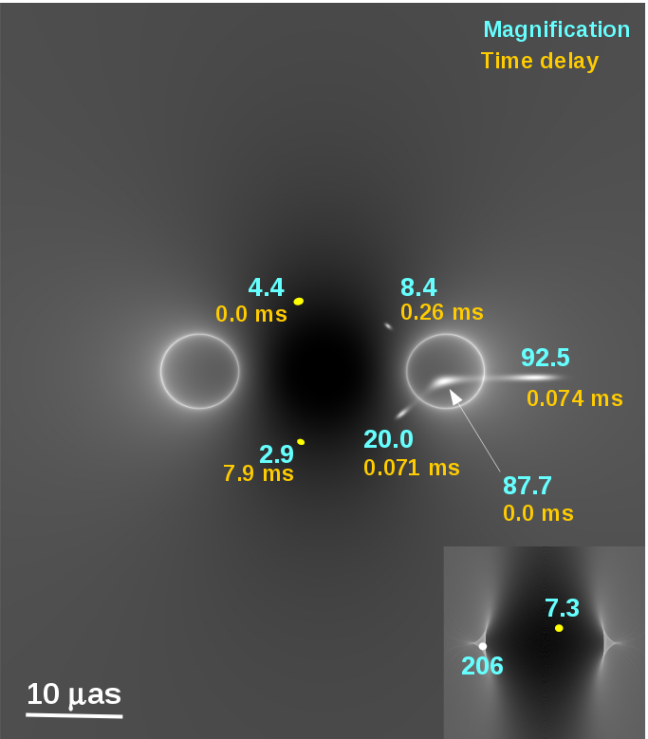
One of the first hints that something unusual was happening for Mothra is that the image we detect with our telescopes corresponds to the one with negative parity. The image with positive parity is not detected. Again, this is still possible for relatively short periods of time (months to a year) since images with negative parity can momentarily have large magnification factors. Howevere, for Mothra, the magnification has remained unusually large for at least 8 years, which is the time spanning since the first Hubble Space Telescope of this star in 2014, and the last observations of Mothra in 2022 made with the James Webb Space Telescope. Stars in the galaxy cluster (or microlenses) can not maintain large magnifications factors for this long period. A much more massive object (a millilens) is needed with a mass at least ten thousand times the mass of the Sun. This millilens needs to be close enough (in projection) to the detected image of Mothra in order to magnifiy it for at least 8 years. The image below shows a cartoon representation of what it is believed to be the configuration near Mothra.

The two blue ellipses represent the double (specular) image of the galaxy hosting the star Mothra. LS1 and LS1′ are the two expected images of Mothra. Only LS1 is clearly detected suggesting that some invisible massive object near LS1 is magnifiying the image of Mothra forming at LS1, but not the other image forming at LS1′ . The black dot represents the approximate position of the halo of DM needed in order to magnify Mothra for at least 8 years. This timescale sets the lower limit of this halo. Masses below 10000 times the mass of the Sun can not magnify LS1 to the required values for 8 years without fine tuning the relative velocities and direction of motion of Mothra. The two red dots represent an unresolved object in the host galaxy that is imaged twice at positions b and b’ (as expected in lensing). These two objects appear with similar brightness in the JWST images, indicating their magnification is not being perturbed by any small structures. This fact is used to set an upper limit on the dark matter halo. It is found that this halo must be at most 2.5 million times the mass of the Sun. Otherwise, the clump c’ would be fainter (demagnified), contradicting the observations. Hence the mass of the black object in the image above is between 10000 and 2.5 million times the mass of the Sun.

This constrain on the mass is used to study possible models of dark matter. One particular model, known as Warm Dark Matter (WDM) predicts that the DM particle is relatively light and moves very fast (almost at relativistic speeds). In this scenario, small structures can not form because the velocity of the DM particle is larger than the escape velocity of the small structure. There is a relation between the mass of the DM particle and the mass of the smallest halo that can exist in this model. From the constrain in the mass of the halo discussed above, astronomers found that models where the DM particle is lighter than 8 keV are in tension with the observations. This is one of the tightest constraints on this type of model coming from astrophysical probes. An alternative model of DM is tested with this observation. It is known as Fuzzy Dark Matter or FDM. In these models, the mass of the DM particle is incredibly small, in the range of 1E-22 eV. Such a small mass has a very large associated De Broglie wavelength (this determines the quantum size) in the astrophysical scale. Lighter masses have longer wavelengths and for very small masses, the wavelength is larger than the size of small galaxies. Since we observe small galaxies around us, this has been used in the past to set a lower limit on the mass of the DM particle for this particular model. From observations of Mothra, one can study the optimal mass range in which the observations would math the predictions from the FDM model. It was found that if the DM particle is in the range of 0.5e-22 eV to 5e-22 eV, the lensing perturbation from the FDM could explain the observations of Mothra (detection of LS1 and non-detection of LS1′). This mass range is interesting because it explains other issues that could be in tension with the standard cold dark matter model, such as the lack of cusps in dwarf galaxies or the possible defficit in the number of satellites around more massive galaxies. Some of these issues can be alleviated with non-exotic baryonic physics but the debate continues about these possible tensions with the standard model. More observations, similar to Mothra, will help in the near future to favor some models against others until we reach the point where only one model survives, or more interestingly, no known model is able to reproduce all observations simultaneously, demanding a new revolution in our understanding of the universe.

Link to the published paper

Eärendel star

Posted on by chemadiegor
Eärendel is found in Norse mythology and Tolkien’s books (morning star).

Eärendel is found in old Norse mythology as a star created by Thor out of one of the toes of Aurvandill. Eärendel was later adopted by Tolkien to refer to the morning star. This reference to an early star is appropriate to talk about Eärendel, the farthest star ever observed.

EärendelThe first stars are expected to form when the universe is between 50 and 100 million years old. Although this may sound as a big number, in reality the universe was still very young at this point. If we compare the universe today (13700 million years old) with a person of 80 years of age, the first 100 million years in the universe would be similar to the first six months of the 80 year old person. That is, still a baby universe. These first stars were expected to be very massive (up to 1000 times the mass of the Sun) and luminous, and composed of basically two elements, Hydrogen and Helium (with traces of Lithium). Given the large size of these stars, they burn very rapidly (like a big fire) and do not live very long (compared with smaller stars like the Sun). After several millions of years these stars die. The death of stars represents one of the most important events for life, since it is then when elements such as Carbon, Oxygen, Iron etc are formed. Eärendel is not one of these first stars, but it could be a star formed from the ashes of these first stars after mixing with more Hydrogen. Eärendel is so far that the light we see from it now started its journey when the universe was still an infant (in Cosmological terms). If we compare again the age of the universe today with an 80 year old person, the light we see today from Eärendel was created when the universe was 5 years old, a universe coming out of its toddler years. The light from Eärendel has been traveling for almost 13000 million years before reaching our telescope (the Hubble Space Telescope to be more precise).

Eärendel and Gravitational Lensing

In its journey toward us, the light from Eärendel has crossed several structures. One such structure is the galaxy cluster WHL0137–08, at approximately 1/4 the distance between us and Eärendel. This cluster is a large collection of galaxies, gas and dark matter, and is so massive that it can bend the space around it. This bending of space makes the light traveling through the cluster to bend as well. The effect is known as gravitational lensing, and is similar to the bending of light when it crosses a dense transparent medium, such as a lens made of glass.

Eärendel is the small dot marked with an arrow. The arc where Eärendel is found is being magnified by a galaxy cluster.

This effect was predicted by Einstein and has been observed many times around very massive objects, like galaxy clusters. The gravitational lensing effect can significantly amplify the light of distant objects, making them detectable with current telescopes. This is exactly what is happening with Eärendel. Without he gravitational lensing effect, we could not have observed Eärendel, but thanks to the amplification from the galaxy cluster, we observe Eärendel thousands of times brighter than what we would have observed without this effect. Other stars at smaller (but still incredibly large) distances have been observed in the past thanks to this effect as well. In the past we have discussed the case of Icarus and more recently Godzilla. All these stars have in common the fact that we are seeing them thanks to the extra magnification provided by gravitational lensing that effectively transforms a relatively small telescope like Hubble into a much larger telescope with a mirror size typically 30 to 70 times larger. Telescopes of this size are impossible to build with current technology, even less if they have to operate from space, but gravitational lensing makes it possible to experience having such a gigantic telescope.

Stars like Eärendel, Icarus and Godzilla are extremely bright and rare but are offering unique opportunities to study the evolution of stars in the earlier epochs of the universe. Future telescopes like JWST working in conjunction with gravitational lenses will push the limits even further and will discover stars even more distant than Eärendel, reaching perhaps the first stars mentioned at the beginning of this post.

Link to research article in Nature and Press releases

A highy magnified star at redshift 6.2

Press release NASA

Press release ESA

Godzilla, a Monster Star

Posted on by chemadiegor
Godzilla is probably a star similar Eta Carinae, but much bigger and brighter.

Big stars are rare but make themselves obvious since they are very luminous. In our Galaxy, there are some massive and superluminous stars. For example Eta Cariane, shown in the illustration above, is a very massive star and among the most luminous in our Galaxy. The explosion-like appearance of Eta Carinae is the result of dramatic episodes in the past, where the star chokes on its own massive energy production and ejects large amounts of matter at great speeds from the outer layers in the star. One such eruption took place in the mid 19th century and is known as the Great Eruption. The eruption did not destroy the star which is still producing large amounts of energy. Other eruptions had taken place in the past. Typically every 300 years Eta Carinae has one of this epileptic episodes. During the last Great Eruption, Eta Carinae become one of the brightest stars in the sky for several years. Even though it is still one of the most luminous stars in the Milky Way, due to its large distance to us, nowadays it appears much fainter than it was in the mid 19th century. It is expected that Eta Carinae will go through another violent episode like the Great Eruption, maybe the last one, hopefully not with a gamma ray burst associated to it and pointing to us, which would jeopardize life here on Earth. But that is another bed-night story…

Today we talk about another star like Eta Carinae, but which is much, much, much farther away. We name this star Godzilla because it truly is a Monster Star. At the moment of writing this post, Godzilla is in fact the farthest star ever observed by humans (that has been published in arxiv). This record will not last long, as in a couple of weeks we will announce the discovery of another star that is even further away (look for a press release by NASA/ESA on March 31st). But is fair to say that Godzilla is the most luminous star we have ever observed, and this record may hold for quite a long time.

Godzilla has been observed with several telescopes, including the Hubble Space Telescope (HST). This star is in the famous Sunburst galaxy, at redshift 2.37, or in layman terms, this galaxy is almost at half the distance (comovil) to the edge of the observable universe (defined by the cosmic microwave background). Alternatively, the light from Godzilla took 10900 millions of years to reach us and the universe was 20% of its age when the light we see today left the surface of Godzilla. Since massive stars are like rock stars (live fast and die young), Godzilla is long dead, but we still see its light since it takes very long to reach us (10900 millions of years as mentioned above).

Godzilla is a unique star for several reasons. It’s luminosity is brighter but still comparable to that of Eta Carinae during the Great Eruption. That is, the light we are receiving now from Godzilla was emitted when Godzilla had an eruption, and temporarily increased its luminosity by a factor ~100 during a period of several years or decades. Since the universe is expanding, if a distant event has a duration of 1 year, when we observe it the duration is increased by a factor (1+z), where z is the redshift (z=2.37 for Godzilla). Hence, if the eruption in Godzilla lasts 10 years in Godzilla’s time, for us the same event will last almost 34 years. This effect is called time dilation, and you can experience it by yourself the next time you go to the airport. When you see one of the moving ways at the airport, walk (or better run) in the direction opposite to where the moving way moves. It will take you longer to reach the other end than if you cover the same distance walking (or running) outside the moving way. For light traveling through an expanding universe, the effect is somewhat similar since as the light travels (at the speed of light) the distance between Godzilla and the telescope increases.

The fact that Godzilla is so luminous is unique in its own way, but that alone would not make Godzilla the special star it is. We estimate there must be millions of stars like Godzilla in the universe but at the distances of Godzilla, these stars would still be too faint to be detected, even with powerful telescopes such as HST. In order to see them, one would need much larger telescopes. Building such enormous telescopes is beyond the reach of current technology but nature can be playful some times, and has offered us a way to emulate gigantic telescopes and tease our curiosity.

Godzilla. King of the Stars during an “episode” of intense activity

A giant natural telescope

Godzilla was discovered thanks to a natural gigantic telescope that happens to be perfectly aligned with our solar system. Pointing a telescope like Hubble towards this gigantic telescope allows us to see what lies behind with incredible resolution. The natural telescope is a gravitational lens, which works thanks to the bending of space predicted by Albert Einstein around massive object. This massive object is a big galaxy cluster with a mass many trillions of times the mass of the Sun. The galaxy cluster can amplify the light of objects which are placed in particular positions behind the cluster, the same way a regular magnifying glass magnifies more the objects which are closer to the central part of the lens. Godzilla happens to be placed in one of this very special locations and we estimate the magnification from the cluster is approximately a factor x3000. When observing with the Hubble telescope, the combined effect of Hubble plus the cluster is similar to having a telescope ~50 times larger, i.e a space telescope with a diameter of 120 meters! The largest telescope on Earth has a diameter of approximately 10 meters, and the largest telescope in space is the new JWST with a diameter of 6.5 meters. The next generation largest telescope planned for the next decade is the ELT with a diameter of 40 meters. The opportunities offered by these natural telescopes, or gravitational lenses, will not be matched by our technology for many decades. Hence they can offer a glimpse of portions of the distant universe with unprecedented detail. Godzilla happens to be in one of these very special positions so we can look through a pinhole of the vast cosmos where nature has placed one of this natural telescopes for us to take a pick.

Godzilla is just the first of many examples of Monster Stars with temporary eruptions that will be discovered through a similar technique. Other stars have been discovered in the past thanks to similar lucky alignments of natural telescopes (see for instance Icarus, the first of such stars) but Godzilla is an even more rare star due to its current activity. Exploiting the possibility offered by natural telescopes, we will soon discover more stars like Godzilla and start to study them in their different phases in order to understand how they evolve and eventually die (sometimes with a very energetic phenomena like a SN).

Link to the research article: https://arxiv.org/abs/2203.08158

Godzilla Poem I, by ChatGPT

In the vast expanse of space,
Where stars and galaxies race,
A creature awakens with a roar,
Its name, the mighty Godzilla.

With scales as tough as titanium,
And a strength that defies quantum,
This king of monsters stands tall,
Ready to fight and conquer all.

But amidst the starry sky,
Something catches its fiery eye,
A starburst cluster of light and heat,
A spectacle that’s hard to beat.

As Godzilla draws near,
He sees something that’s not quite clear,
A lens that warps the star’s bright flare,
A cosmic wonder beyond compare.

The monster gazes in awe,
At this breathtaking scene he saw,
A reminder that in the grand scheme,
Even he’s small, just a single beam.

And so Godzilla moves on,
His heart filled with wonder and song,
For even a creature so grand and bold,
Can still be humbled by the universe’s hold

Godzilla Poem II, by Bard

Godzilla star,

Lens of the Sunburst galaxy,

So bright and far.

Your light travels through time,

Reaching us from 10.9 billion light years away.

We are but a fleeting glimpse of your past.

Your sunburst glows,

A beacon of hope in the darkness of space.

We are drawn to your light, Like moths to a flame.

Godzilla star, We are grateful for your presence.

You remind us of the vastness of the universe,

And the beauty that can be found in the darkness.

The importance of Prior-itizing

Posted on by chemadiegor

The quest for lensed gravitational waves

We are all subject to personal biases, even when we try not to be biased. In Science, a popular tool to select among a variety of models is inspired on Bayesian theory. According to this theory, the most likely model is the one that offers the best description to the current data (i.e fits the data), and given by its likelihood, but also is consistent with previous knowledge on some of the parameters that are being fitted. Previous information is accounted for by a term known as the prior. Good priors result in fairs results. Biased priors often lead to the wrong conclusion.

In a recent paper, the LIGO-Virgo collaboration (LVC) studies the possibility that gravitational waves (GW) are being strongly lensed. If lensing is taking place, a fundamental prediction from gravitational lensing theory is that multiple occurrences of the same GW should take place, with a time separation between them of typically days to months. LVC presents a series of candidates which show high consistency with the lensing hypothesis, or in the Bayesian terminology, have a good likelihood of being pairs of images of the same GW. All these pairs are later discarded as possible lensed images based on the prior term, that heavily penalizes pairs of GWs separated by more than a few weeks. They finally concluded that lensing of GWs is unlikely, based on their final score.

Distribution of time delays of observed lensed quasars (orange) and gravitational waves (red)

This is however a conclusion that is obtained after adopting potentially bad priors. The LVC does not provide sufficient information regarding their prior, but in a recent study we show how the prior used by LVC is in tension with observations of time delays from real gravitationally lensed pairs of images. In fact, the distribution of time delays between the pairs of gravitational waves which LVC found to be good candidates to be strongly lensed, is consistent with the known distribution of time delays from quasars and from analytical models.

LVC finds that approximately half the events published in the O3 catalog can form pairs of lensed GW events (that is, there is another GW which shows high consistency in terms of GW parameters and sky localization, as predicted by lensing). As shown in the image accompanying this post, the separation in time between the two GWs forming the pair (solid red curve) is consistent with the known distribution of time delays (dashed orange curve). In the LVC analysis, pairs of GWs with time separations of approx 4 months are directly assigned a probability of zero, contradicting real observations where such time delays are possible. LVC concludes that none of their candidates to be pairs of gravitationally lensed GWs favours the lensing hypothesis, but this conclusion is biased by the adoption of a prior that intrinsically negates the possibility that realistic time delays are possible.

The use of a better (unbiased) prior does not necessarily mean that the opposite conclusion is true (ie. that lensing is favored), but it begs the question of whether the conclusions from LVC could have been reversed.

If you want to find more about this discussion you can check our latest work, or our earlier work where we present a model that predicts that gravitational lensing of GWs has already been observed.

The Kaleidoscopic Universe

Posted on by chemadiegor

In very few scientific fields, color matters more than in Astronomy. First photographic plates, and later CCDs, capture the light of distant objects by integrating during long exposures. When photons hit the CCD, small electric currents record the light intensity. Current CCDs working in the optical, UV and NIR range are basically insensitive to the “color” of the arriving light and filters need to be placed in front of the CCD to select the spectral range, or color, that wants to be studied. Often, these filters are “wide” in the sense that they allow a generous amount of light to go through (since distant objects tend to be faint), and the entire optical spectrum can be covered with 3 filters. However, their are situations where much narrower filters would be desirable. Astronomical objects tend to emit light in a combination of continuum (free-electrons being captured by the atoms) and spectral lines (electrons jumping between atomic levels). The spectral lines carry very valuable information about the conditions (like temperature and metallicity or abundance of elements heavier than Helium) that get diluted when observing the objects with wide bands. On the other hand, sufficiently narrow bands can resolve these spectral features.

Today (July 7th 2020) we published the first results of the J-PAS survey pathfinder (or miniJPAS). J-PAS is a narrow band survey of 15% of the sky, with an unprecedented number of narrow band filters (54). The image below shows the 54 narrow-band filters, plus 5 of the wide-band filters.

Using the narrow-band filters, J-PAS can identify features as the H-alpha line, or the 4000 Angstrom break, allowing for precise SED fittings and photometric redshifts of all galaxies in the catalog.

J-PAS will be particularly powerful at detecting rare objects with string emission lines. Chief among these, high redshift quasars, where the bright Lyman-alpha gets redshifted into the J-PAS spectral range, allowing for unambiguous estimation of the quasar redshifts. Earlier estimations suggest that J-PAS will be capable of detecting half a million quasars in the surveyed area.

The superior power of J-PAS to estimate photometric redshifts will open the door also to do tomographic studies and unveil the 3D structure of the galaxy distribution to unprecedented detail. Stay tunned for more. First light of the full scientific survey is expected to take place in late 2020 or early 2021. You can also find the first data release in J-PAS website and explore the images directly from your browser using the Sky Navigator

You can find the paper below and in this link https://arxiv.org/pdf/2007.01910.pdf

Click to access 2007.01910.pdf

Is LIGO really seeing Mass Gap events?

Posted on by chemadiegor

The recent publication by LIGO of two events (GW190412 and GW190814) with high mass ratios, and with one of the masses close to the mass gap (that is, a mass between 3 and 5 solar masses which are difficult to explain with standard models) has created an intense debate on the nature of these objects. If confirmed, the implications of these observations are important since they can give us information about the equation of state of neutron stars (where one can study exotic forms of matter, such as axions or hyperons), or reveal a new type of black hole, including a leading dark matter candidate, primordial black holes.

However, a simpler alternative could be that these events are strongly lensed. Gravitational lensing can amplify the signal of observed gravitational waves, allowing their observation from much farther distances (and hence much larger volumes). In earlier work, we showed that if the rate of mergers (that produce gravitational waves) at redshift z>1 is sufficently high, observation of these distant events by LIGO is not only possible, but unavoidable. On fact, for rates larger than a few times 10^4 mergers per year and Gpc^3, lensed events will dominate over not-lensed events, in a similar fashion as lensed gravitational lensed IR galaxies dominated over not-lensed IR galaxies in the bright end of Herschel observations.

The left plot shows the prediction from our lensing model (colored circles) compared with the observations (squares and diamonds with error bars). All events concentrate around two locus regions. BBH and NSBH. Note how observations match perfectly the prediction.

 

Lensed gravitational waves get stretched due to cosmic expansion as they travel from their originating source to the detector. The farther the source is, the lager the stretch. The stretch is proportional to (1+z), where z is the redshift if the source. Higher z translates into gravitational waves that, when observed, appear as having a longer wavelength. If the gravitational wave is being magnified by strong lensing, and this magnification goes unnoticed (there is no way a priori to know if a gravitational wave is being magnified), the longer wavelength will be missinterpreted as being due to a larger mass of the two compact objects that ar causing the gravitational wave. For instance, if a neutron star with a mass of 1.3 solar masses is merging with a black hole of 12 solar masses (these are the typical masses found in our Galaxy for these objects) at redhift z=1, the observed gravitational wave will appear identical as the one from a much closer merger (z =0) with a neutron star of 2.6 solar masses and a blackhole with 24 solar masses. This example is not arbitrary since it was chosen to match the observed masses of the latest published gravitational wave event , GW190814, interpreted as being a local event (z=0), but which based on our interpretation could be also a lensed event at z=1 or z>1.

The lensing model interpretauon makes a series of interesting predictions, but among these, it is interesting to pay attention to the predicted mass ratio for binary black holes and neutron star black hole mergers. As shown in the figure illustrating this blog, lensing predicts these type of events will appear in the M1-M2 plane in two well defined regions. Interestingly, all observed data points so far agree remarkably well with this prediction. The two possible mass gap events are marked with a big yellow circle and follow well the predicted locus for lensed NSBH events. If confirmed, our lensing model would offer a simple solution to the mass gap problem, and would imply a much higher rate of events at z>1 that previously thought. 

You can see our full work below. A Fun Fact about this paper is that it was put “On Hold” by arxiv moderators after being submitted to arxiv on June 19 (Friday). After requesting an explanation from arxiv, none was given. At the time of our original submission, we where unaware of the upcoming publication, by the LIGO team, of the GW190814 “Mass Gap” event. The following week, in June 24 we saw in arxiv the LIGO paper with the values of M1 and M2 for GW190814. The same day, the “Hold” on our paper was lifted and we where able to just add the new data point to our figure (see point marked GW190814 in the figure above), before it appeared on arxiv the following day (June 25). I do understand (and support) the need for moderation in arxiv, but this process is far from transparent. The lack of communication and explanation of why papers are being put on hold, inhebitably leads to one suspect foul play, which is something that should be avoided at all cost, specially in portals such as arxiv, that makes research freely available. 

 

Link to paper https://arxiv.org/pdf/2006.13219.pdf

Click to access 2006.13219.pdf

Seeing through Dark Matter with gravitational waves

Posted on by chemadiegor

We covered the topic of dark matter before in this post (Dark Matter under the microscope). Dark matter remains one of the bigegst mysteries of Science. One of the candidates for dark matter are Primordial Black Holes or PBH. PBH are black holes that formed during the first instants of the universe. Like dark matter, PBH do not emit light and interact with the rest of the universe basically only through gravity. The LIGO experiment has been detecting a surprisingly high number of massive black holes. The origin of these black holes is uncertain but one of the possibilities is that they could be PBH. We also discussed LIGO detections in this earlier post (Did LIGO really see massive black holes?) . In order to explain the current observations by LIGO, only a fraction of the dark matter needs to be in the form of PBH. In particular, a fraction as small as 1% of the total dark matter would be sufficient to explain the unusually elevated rate of black hole mergers with masses above 20 solar masses.

In a new work we discuss a novel method to explore the possibility that PBH constitute part of the dark matter. Our latest paper (see link at the end of this post) studies for the first time the interference produced when gravitational waves cross a portion of the sky populated with a realistic distribution of stellar bodies (stars, neutron stars or black holes) or microlenses. Earlier work have considered only the simple, but unrealistic, case of isolated microlenses and at most assuming that they are located near a larger lens (galaxy or cluster) but always on the side with positive parity (a tecnicallity that describes one of the two possible configurations for a lensed image). Our work goes further than these simple exmaples by studying the combined effect produced by a realustic population of microlenses and also considers the unexplored regime of macroimages with negative parity (they constitute roughly half  the images produced in the string lensing regime). The figure accompanying this post shows an example of a single microlens embeded in a macrolens and on the side of the lens plane with negative parity. The numbers in orange represent relative time delays (in milliseconds) between the different microimages (the numbers in white indicate the magnification of each microimage and the grey scale shows the magnification in the lens plane with the critical curves shown as two white circular regions. The inset in the bottom-right shows the corresponding magnification in the source plane with the position of two sources, one white and one yellow). At LIGO frequencies (approx 100-500 Hz), a time delay between 1/500 seconds or 1/100 seconds (that is or 2 or 10 milliseconds  respectively)  can produce constructive or destructive interference in the incoming gravitational wave at the detector. For the example in the figure, the microlens has a mass of 100 solar masses. These type of masses where known before to be capable of producing such interference but what our work show is that the mass can still be significantly smaller (a few solar masses) provided several microlenses can work together to produce time delays of order several milliseconds. This cooperative behaviour takes place naturally when one is observing gravitational waves that are being lensed by large factors (of order 100 or more) since in this case, two microlenses which are relatively distant from each other in the lens plane, can overlap their regions of high magnification (known as caustics) in the source plane, if the magnification from the macromodel (galaxy or cluster) is sufficiently large (in a fashion similar to how a magnifying glass works that can bring photons that are separated by some relatively large distance to come together at the focal point of the magnifying glass). Our study shows that interference of a gravitational wave with itself due to microlenses is not only possible, but unavoidable if the magnification from the macromodel is sufficiently large.

This result opens the door to constrain the abundance of PBH. If PBH are as abundant as 1% of the total dark matter, the interference signal observed in detected gravitational waves here on Earth would be significantly different. Next in the list is to study by how much we can constrain this abundance as a function of the mass function of the PBH. Stay tunned …

Preprint to the science article

A Universe of extremes

Posted on by chemadiegor

 

Caustics_Zoom10_Spera_50muasx625muas_ROT

Ever wondered how space looks like? This apparently silly question is actually one of the most fundamental questions in Science. Space (and time), the framework where everything we experience happens has some unknown structure on its most fundamental scale which we do not understand yet. On larger scales, the General Theory of Relativity tell us that space time and matter are closely interconnected.   One of the most revolutionary concepts of the 20th century was Esintein’s realization that the geometry of space is determined by the energy content of the Universe.

Thus, a very massive object can curve space around itself. If the object is massive enough, the curvature of space around it can be large enough so it can be measured. Nowadays, the curvature of space has been measured on scales ranging from planet solar system scales to the size of the observable Universe. On the largest scales, the Universe appears as having very small or no curvature. This is what one would expect if the size of the Universe were much larger than the size of the portion of the Universe we can actually observe. Think of yourself at a boat in the middle of the ocean and with a clear view of the horizon. If you measure the curvature of your portion of the Universe (that is, everything around you up to the horizon) you will conclude that the space is flat but if you were able to jump on a spaceship conveniently stored in your boat you will see that as you go higher and higher you can start to see the curvature of Earth. In a similar fashion, our limited view of a small portion of the Universe makes us erroneously believe that we live in a  flat Universe.

On the other hand, if you were to look from your boat not at the horizon but at the surface of the water, you would see waves in the water and conclude that on small scales, the water (the Universe) is not flat but it has regions with positive curvature and regions with negative curvature.  Similarly, the Universe on small scale has ripples in space, like the waves, that can be measured. But how?

Imagine now that you jump from the boat and go scuba diving. In your journey to the bottom of the  Ocean you look up and see a bright spot of light, the Sun. But the Sun looks different now. It does appear  to fade for an instant and then get brighter, and it repeats the same pattern of fading and brightening,  again, and again, and again.  This change in brightness of the Sun is a consequence of the curvature of the water (the waves) that, as they move on the surface, act as lenses focusing the light into your eyes during a small instant where you see a brighter Sun and one instant after the focus moves away from your eyes so you see the Sun fade. You have to wait for another wave to align with your eyes so they focus again the light into your eyes and so on. In theory, you could learn a lot from the waves by simply looking at the change in brightness of the Sun. By measuring how many times the Sun brightens every minute, you could estimate the number of waves (and their speed) that pass through a given point per minute. By measuring how much brighter the Sun is during a maximum than during a minimum,  you could say something about the shape of the waves. Are they tall waves that give you big differences between the maxima and minima or are they small waves where the change in flux is much smaller?

The curvature of space can be measured in a similar way. By looking at a bright distant object, if a massive object (a lens) moves between us and the bright object, it will curve the space around the massive object, the same way the wave curves the surface of the Ocean. The massive object will act as a lens (we call them gravitational lenses since the curvature of the space is proportional to the gravitational force of the object acting as a lens) and the bright object may brighten or fade as the massive object moves between us and the bright object. This technique is known as gravitational lensing. By measuring the change in brightness of the bright object we can learn things about the massive object acting as a lens. In particular, we can estimate the mass of that massive object.

Now, this is where things become more interesting. Having a reliable method to measure masses of distant objects is (one of ) the holy grail(s) in Astrophysics in general, and Cosmology in particular.  One of the biggest mysteries of Science (actually the biggest for some of my colleagues) is to understand the nature of dark matter. What is it made of? Is it even real or just a (huge) flaw in our models? One candidate for dark matter, which is  discussed in some of my earlier posts, is Primordial Black Holes (see this post for instance) or PBH for sort. If PBH account for a significant fraction of dark matter, they should be producing ripples in space that could be measured using the gravitational lensing technique mentioned above. In particular, as discussed in the post mentioned earlier, PBH with masses about 20 to 50 times the mass of the Sun are particularly interesting 1) because this is pretty much the only range of masses where PBH have not been excluded yet and 2) because LIGO is finding a surprisingly large  number of PBH in this mass range. Could it be that dark matter is made (at least part of it) of PBH? If enough PBH are moving around in the Universe, occasionally they may align with a bright object in the background so  the gravitational lensing effect would produce a change in the brightness of the object that could be used to measure the mass of the PBH. The chance of these alignments is however very small and it is very unlikely to witness one of these alignments (they can last a relatively sort period of time of a few days or weeks). However, we, astronomers, are great at gambling though, because we always cheat.

Instead of waiting for one of these rare alignments looking at a random bright object far away, we can search for these alignments in a place where we know the odds of observing one alignment are much bigger. These places are the caustics of large lenses, generally groups of galaxies or clusters of galaxies. In the Ocean analogy, imagine that while you are scuba diving and looking up at the Sun, a Tsunami moves on the surface of the Ocean. The Tsunami creates a giant wave extending over hundreds of meters. The giant wave acts as a giant lens collecting light from hundreds of meters and focusing all the light into a narrow line  that moves together with the Tsunami.This line is called a caustic (for its similarity with the caustics produced by regular lenses). When this line (or caustic) passes by you, you will see the Sun much brighter (in fact, it might blind you) than when the much smaller waves were focusing the lights. But if you look closer, you will see that the caustic is not a perfect line. Instead, the surface of the Tsunami is not perfectly smooth but it still contains small ripples (or microcaustics) caused by the smaller waves riding the Tsunami. If you count the number of microcaustics that travekl surrounding the big caustic of the Tsunami, you will find that the density of microcaustics is very high. The Tsunami is concentrating also the light from all the ripples (microcaustics) extending hundreds of meters into a small region near the caustic of the Tsunami. All the sudden, the odds of having one of those microcaustics aligning with your eyes is much higher when you look at the Sun through the Tsunami. In a real observation, the Tsunami would be a very massive object like a group or cluster of galaxies and the small waves, or ripples, would be the PBH (or other type of small objects like regular stars, stellar black holes or neutron stars). In 2016 we witnessed the first example of an alignment of a very bright star at a cosmic distance being magnified by a star or black hole in a galaxy cluster (see this previous post). Only a few days ago (June 5 2018) the same star went through another ripple and we expect more in the near future. By measuring the frequency of these alignments and studying in detail the way the brightness of the star changes as it moves through the ripple we expect to learn more about dark matter or at the very least, put a cross in another model as an invalid one.

The image accompanying this post shows a crowded field of microcaustics at the position were the caustic of cluster would be if there were no microlenses. If microlenses (PBH for instance) were not included in this simulation, there would be simple a horizontal bright line, the  caustic of the cluster of galaxies. In a recent paper we discuss this result in detail and estimate the probability of having this type of alignments using as bright background objects supernovae, very bright stars including Pop III stars and gravitational waves.

Follow this link to read the paper

Did LIGO really see massive black holes?

Posted on by chemadiegor

gravitational-waves-18
GW being emitted by a pair of black holes

The LIGO (and now Virgo) experiment has opened a new window to explore one of the most mysterious objects in nature, black holes (BH). When two black holes merge, they create a cataclysmic event that sends waves through the fabric of space itself and can travel cosmic distances. This is similar to an earthquake shaking Earth. These waves are known as gravitational waves (GW) and until 2015 they were just pure speculation as no experiment was ever able to detect them. Despite the tremendous amount of energy released when two BH merge (a binary BH merger), these waves, or ripples in space-time, are incredibly difficult to observe. The distortion  that a binary BH merger  in a nearby galaxy induces in space-time is minuscule when it reaches Earth. So minuscule that LIGO need to measure tiny shifts in the relative position between two mirrors which are several orders of magnituude smaller than the size of the smallest atom. This is an incredible achievement.  LIGO’s first detections of GW have brought a few surprises though. And they started with a bang!

The first event detected by LIGO in 2015 was interpreted as a heavier than expected binary BH merging in a  closer than expected galaxy. Similar events have been observed since raising several questions. Are these events more common than previously thought? Why have not we see them farther away if they are stringer than expected?  The mass of the individual black holes forming the binary BH were inferred to be approximately 30 solar masses each. Note that I say inferred because these masses could not be measured directly. What LIGO can measure with relative high precision is what is known as the observed chirp mass.  The intrinsic chirp mass is some combination of the two masses of the binary black hole. If both masses are similar, the chirp mass is similar to those masses as well. If the two masses of the binary BH are very different, the chirp mass will be a value in between these two masses (but closer to the mass of the lightest component). The observed chirp mass is related with the intrinsic chirp mass by the factor (1+z) where z is the redshift of the binary BH. The redshift is a measure of the distance so more distant objects have a larger redshift (our redshift is zero).  In other words, what LIGO can measure with good precision is Mo=Mc*(1+z) where Mc is the intrinsic chirp mass. Mo determines the frequency at which the GW is oscillating, a number that LIGO can estimate quite well. For that very first event, LIGO found that Mo had to be approximately 30 solar masses and that the distance was relatively small, that is z was close to zero. Hence, the intrinsic chirp mass (and mass of the individual BH before they merged)  had to be also close to 30 solar masses. This came as a surprise since many predictions made years earlier anticipated that such high values for Mo should be very rare. In fact, what was expected was to find values for Mo between 7 and 15 solar masses. This was in part motivated by observations of X-ray binary stars in our Galaxy, for which  it is possible to estimate the mass of the BH. An X-ray binary is a pair of closely orbiting  objects where one is a star and the other one is either a neutron star or BH. In this article we consider only the BH case. Roughly speaking, by measuring the amount of light emitting by the gas (from the star) spiraling towards the BH one can measure the mass of the BH.  In our Galaxy, the mass of about a dozen BH has been measured using this technique. The results show that the BH masses are between ~7 and ~14 solar masses. So far, no BH with a mass higher than 20 solar masses has been found in our Galaxy raising another, even more fundamental, question. Is our Galaxy special or is there something else we are missing regarding the BH masses and distances inferred by LIGO?

This is the question we address in our latest work. Owing to the degeneracy with the redshift described above, would it be possible that the intrinsic chirp mass was smaller if the redshift was higher? If the redshift is, let’s say z=1, instead of z~0 then, the intrinsic chirp mass could be a factor of two times smaller than the value inferred by LIGO (while keeping the observed chirp mass constant) bringing it into agreement with the BH masses observed in our Galaxy. There is one caveat though.  If the GW was originated in a galaxy far away at redshift z=1, instead of in a galaxy nearby (z~0), the intensity of the GW would have been much smaller than what LIGO observed. The intensity of the GW  is (to first order) the quantity that is used by LIGO to determine the distance. The observed intensity determined then that the inferred distance had to be relatively small. A mere few hundred Mpc instead of several thousand Mpc which would be the distance for a galaxy at redshftz~1 so one would conclude that the GW originated in a nearby galaxy and consequently, the intrinsic chirp mass had to be high. But this is the funny thing. Nature has interesting ways of playing with us. One of these ways is gravitational lensing thanks to which, an object that is far away may appear to us as if it were much closer (that is, it can amplify the intensity mentioned above). Note that I used the expression infer again when referring to the distance estimation by LIGO. This estimation is made under the assumption that gravitational lensing is not intervening. This is normally a good assumption since, after all, only a very small fraction of distant objects get (significantly) affected  by gravitational lensing. To be more precise, 1 in approximately 1000 or 10000 objects at redshifts larger than z=1 are substantially magnified by the gravitational lensing effect. Hence, is it still possible that a significant fraction of the LIGO events are distant lower mass events that are being magnified by gravitational lensing?  In our work we find that lensing can just make the trick. At large distances, the volume of the universe that is reaching us now (and by this I mean the volume where the light or GW we see now originated) is much  larger  than the corresponding volume at much smaller distances. To visualize this, imagine the volume of a shell of radius R. This volume goes like the square of the radius. So a large shell with a radius 10 times larger than a smaller shell will have 100 times the volume (if they both have the same thickness).  By precise calculations of the gravitational lensing effect over distant gravitational waves we prove that the massive and nearby events found by LIGO can in fact be interpreted as normal but more distant events with masses comparable to the ones found in our Galaxy. This solves the puzzle mentioned at the beginning of this article. Is our Galaxy special? And if it is not, where are the masses that LIGO claims is finding in nearby galaxies? The answer is that those masses would be the same in our Galaxy and in other galaxies. What is wrong is the interpretation of the observation since the amplification due to lensing has been ignored (this story is very similar to the puzzling first bright galaxies detected by Herschel that turned out to be all gravitationally lensed distant galaxies) .

So why has not anybody realized this earlier? That is a good question and the answer is not because people have not thought about this before. For our model to work, there is one little thing that sets our study apart from other similar attempts. As we mentioned earlier, at z~1, only one in a few thousand events could be magnified substantially by gravitational lensing. On the other hand, by observing more distant objects one is observing a larger volume, so one is observing more events. The gain in volume with respect to nearby distances is in the range of two orders of magnitude (more precisely about 1.5 orders of magnitude between z=0.1 and z=1 for a shell of thickness dz=0.1). This gain in volume is not enough to compensate the small probability of lensing at z~1 (1/1000 or less). A significant rate of lensed  events (enough to explain the rate of observed events)  can be obtained ONLY IF (and this is the little thing)  one increases the rate of intrinsic mergers at z=1 with respect to the rate at z=0. Such evolution in the intrinsic rate is expected and has been considered in the past. Our study shows that in order for the lensing mechanism to work and be able to explain the LIGO observations (with the troubling masses), the rate at z=1 needs to be more extreme than previously considered. This is not necessarily a problem since we simply don’t know what this rate is and also there are models that predict such rapid evolution in the intrinsic rate of events between z=1 and z=0 but, surprisingly, this type of strong evolution models were not considered in the past so the role played by lensing  was not recognized.

So then. Are we right? Are we wrong? Time will tell. After all, only one (if at all) of the many interpretations proposed to explain the LIGO massive events will be the correct one. An important aspect of any model is that it needs to be testable and this one is. If lensing is the culprit, at high magnifications one would expect a pair of images with similar magnifications and with a small time delay between them (hours to days depending on the lens mass, lens distance and relative source-lens-observer position).  LIGO detections don’t come in pairs (at least no such detections have been reported yet). If the time delay is several hours or days, it is possible that one of the two lensed events falls below the detection threshold of LIGO since the visibility (determined in part by the geometric factor in LIGO, a technicality whose explanation is beyond the scope of this article) may have changed substantially.  For simplicity, we can say that an event that is directly overhead the detector results in a significantly stronger signal-to-noise ratio than the same event near the horizon. Since Earth rotates once every 24 hours, a position in the sky (like the Sun for instance) can move from the zenith to the horizon in six hours. Hence, two identical GW originating in the same spot in the sky may have significantly different signal-to-noise if they are separated by approximately six hours. There is however a limit for how many times you may get the unlucky configuration that permits to hide one of the two images. Eventually two events should be observed that have virtually the same observed chirp mass and a distance estimate that is consistent with the uncertainties introduced by the geometric factor. The ratio of signal-to-noise between the two events should be compatible with the angle rotated by Earth during the time separation between the two events. Finally, the inferred location in the sky (derived from the time difference between detections in different observatories) should be also consistent with being the same for both events. Data mining of the LIGO data may unveil some of these missing events in the near future and confirm the lensing nature of the massive LIGO events.

Link to the publication

You can download the paper with our study in this link

 

 

 

 

 

 

Dark Matter under the microscope

Posted on by chemadiegor

Caustic_ColorDark matter remains one of the main unsolved problems in modern physics. Despite the growing evidence for its existence coming from astronomical observations, all efforts to detect it in a lab on Earth have failed. One possible candidate for dark matter that can not be detected on Earth (and let’s hope it stays like that) are primordial black holes (or PBH). This type of black hole was created during the first moments of the universe and may have survived till today. PBH are invisible (they don’t emit light, or extremely low amounts  if they are not very massive) and pretty much interact with the rest of the universe only through gravitational forces. This is basically the same behaviour as dark matter. Most types of PBH have been already ruled out but they can still exist in certain mass ranges (also, high spin PBH may not have been considered in detail in previous studies and may be harder to exclude). One of these possible  mass range is about 30 solar masses (think LIGO) and the second one is around the mass of a brown dwarf or a planet. A new type of observation may be able to prove these masses and rule out the possibility that PBH could be a sizeable fraction of the dark matter. This observation relies on caustic crossing events like the Icarus and Iapyx events observed in the galaxy cluster MACS1149. The interpretation of these events is that a very distant and luminous background star (z=1.55) is moving in a region that lies very close to  one of the caustics of the cluster (a caustic is a position which results in a large fraction of the light emitted from the star being focused to us at the focal point of the gravitational lens). As it moves, the light of the star gets amplified by the effect known as gravitational lensing. In its path to us, this light passes near stars (microlenses) in the galaxy cluster and the magnifcation changes depending on the distance to  the microlenses. Caustics are normally assumed to be smooth curves. In the presence of microlenses, caustics are disrupted like in the figure accompanying this post that shows a caustic being blown up by many PBH, each with 30 times the mas of the sun (without the PBH the caustic would resemble a single straight line instead of the web shown in the figure). We have studied this new type of observations and shown that through continuous monitoring of caustic crossing events it is possible to constrain the fraction of dark matter in the form of microlenses. So far, preliminary results do not favour an scenario where even a modest fraction of the dark matter ( a few percent) can be made of massive PBH (~ 30 solar masses).

You can read the scientific papers in the links below.

Observation paper

Theory paper

 

Seen stars in motion

A wise man said once that ; “A picture is worth a thousand words“. The wiser man replied, “A movie is worth a thousand pictures“. The movies below show a few examples on how the flux of the background star would change as the star moves across the field of microcaustics in the cases where only stars (and remnants) in the cluster act as microlenses and in the case where 1% of the dark matter is in the form of PBH with 30 solar masses each. For the first four movies the star is made unrealistically large in order to better see the effect (R=70000 solar radii). The magnification does not show large fluctuations as a consequence of this extreme radius.

Video 1) Icarus event with ICL stars

Video 2) Iapyx event with ICL stars

Video 3) Icarus event with ICL stars and 1% dark matter as PBH

Video 4) Iapyx event with ICL stars and 1% dark matter as PBH

An even higher resolution of the effect can be found in the two videos below where the resolution is increased by a factor ~30 and a more realistic star with 1000 Rsun is considered star (this is a typical radius for a giant star) . The first movie considers the more likely scenario where the direction of motion of the star with respect to the cluster caustic is at an angle. The movie considers an angle of 30 degrees but the result would be very similar at any angle larger than few degrees. The second case considers the special case (unlikely) where the motion of the star is aligned almost perfectly with the direction of the cluster caustic. In this case the star approaches the caustics through the cusps of the caustics producing a different pattern in the magnification. The caustic map is shown in the right panel of the movie with the position of the background star shown as a cross. For these movies we only consider microlenses from the intracluster medium (i.e, no PBH) and the central microlens has a mass of one solar mass.

Video 5) Star travelling at an angle with the caustic.

Video 6) Star travelling parallel to the caustic.

Similar movies but with just one microlens can be found in the two links below.In tehse movies, three nearby background stars cross the same caustic from a single microlens having M=1 Msun. The movies show how the same microlens can produce very different magnification patterns depending on the trajectory of the background star.

Video 7) Zoom in on Icarus side. Three stars travelling at an angle with a single microcautic from a microlens with M=1Msun

Video 8) Zoom in on Iapyx side. Three stars travelling at an angle with a single microcautic from a microlens with M=1Msun

 

You can read the scientific papers in the links below.

Observation paper

Theory paper